Textbook solution for College Algebra (MindTap Course List) 12th Edition R David Gustafson Chapter 61 Problem 5SC We have stepbystep solutions cocept of elimation method The sum of the digits of a two digit number is 12 The number obtained by interchanging Its digits exceed the given number by 18 find the number pxqy=pq qxpy=pq x2y=5 3x/23y=10 solve by elimination method RichLets's say 7x15y=2 (eqn 1) x2y=3(eqn 2) let's multiply eqn2 by 7 to eliminate the x variable of eqn 1 Eqn 2 => 7x14y=21 Now subtract eqn2

How Do You Solve X 2 Y 3 6 And X 3 Y 2 12 Socratic
X-y=3 x/3+y/2=6 by elimination method
X-y=3 x/3+y/2=6 by elimination method-Solve for x and y using elimination method xy/xy=2 xy/xy=6 Hi, you may find many different solutions but this is the simplest but check the answer firstQuestion System of Linear Equations Read and solve for the values of the unknown using the suggested method show the solutions 4By Elimination method x y = 3




X 2 2y 3 1 And X Y 3 3 Find X And Y Values Using Elimination And Substitution Method Youtube
The elimination method of solving systems of equations is also called the addition method To solve a system of equations by elimination we transform the system such that one variable "cancels out" Example 1 Solve the system of equations by elimination $$ \begin{aligned} 3x y &= 5 \\ x y &= 3 \end{aligned} $$Question 1 Solve the following systems of linear equations by Gaussian elimination method 2x − 2y 3z = 2, x 2y − z = 3, 3x − y 2z = 1Click here👆to get an answer to your question ️ Solve for x and y x y = 3 4x 3y = 26
For solving pair of equation, in this exercise use the method of elimination by equating coefficients ` x y /6 = 2( 4 x )` 2x y = 3( x 4 ) Advertisement Remove all ads Solution Show Solution ⇒ x = 3 ∴ Solution is x = 3 and y = 9 Concept Methods of Solving Simultaneous Linear Equations by Elimination Method Method of2y = 2 2y = 2 y = 2 1 See answer wandawhite455 is waiting for your help Add your answer and earn points0 votes 1 answer Solve for x and y 10/xy 2/xy = 4 ,15/xy9/xy = 2,where x ≠ y, x ≠ y
Solve by Addition/Elimination xy=3 , xy=7 x y = 3 x y = 3 , x y = 7 x − y = 7 Multiply each equation by the value that makes the coefficients of x x opposite x y = 3The elimination method for solving linear systems Another way of solving a linear system is to use the elimination method In the elimination method you either add or subtract the equations to get an equation in one variableAnswer to Solve the system of equations by elimination method 3 x 2 y = 2 4 x y = 3 By signing up, you'll get thousands of stepbystep



Solve The Following Pairs Of Linear Equations By The Substitution Method 3x 2 5y 3 2 X 3 Y 2 13 6 Sarthaks Econnect Largest Online Education Community




X 2 2y 3 1 Amp X Y 3 3 Solve By Eliminations Or Substitution Method Brainly In
Solve using the elimination method Show your work If the system has no solution or an infinite number of solutions, state this 2x 6y = 12 x 3y = 3 3 Solve using the elimination method Show read moreTextbook solution for College Algebra (MindTap Course List) 12th Edition R David Gustafson Chapter 61 Problem 6SC We have stepbystep solutions Click here 👆 to get an answer to your question ️ xy=3,x/3y/2=6 elimination method 1 Log in Join now 1 Log in Join now Ask your question riddhi riddhi Math Secondary School 5 pts Answered Xy=3,x/3y/2=6 elimination method 2



Elimination Method Simultaneous Linear Equations Siyavula




X Y 3 X 3 Y 2 6 Elimination Method Brainly In
Gió's method uses the method of substitution Here's how to do it by the method of elimination Step 1 Enter the equations 1 #3xy=1# 2 #xy=3# Step 2 Add Equations 1 and 2** #4x=4# 3 #x=1# Step 3 Substitute Equation 3 in Equation 2 #xy=3# #1y=3# #y=2# Solution #x=1,y=2# Check Substitute the values of #x# and #y# in Equation 1 Transcript Ex 33, 1 Solve the following pair of linear equations by the substitution method (i) x y = 14 x – y = 4 x y = 14 x – y = 4 From equation (1) x y = 14 x = 14 – y Substituting value of x in equation (2) x – y = 4 (14 – y) – y = 4 14 – y – y = 4 14 – 2y = 4 –2y = 4 – 14 –2y = –10 y = (−10)/(−2) y = 5 Putting y = 5 in (2) x – y = 4 x = y 4 xThe given equations are `x/3 y/4 =11`(i) `(5x)/6 y/3 = 7` (ii) From (i) we get `(4x 3y)/12 = 11` => 4x 3y = 132 (iii) From (ii), we get




Systems Of Equations With Elimination X 2y 6 4x 2y 14 Video Khan Academy



Chapter 8 Problems
Answer to Solve by the elimination method 3x y = 1;X 2 2y 3 1 And X Y 3 3 Solve By Elimination Method Brainly In For more information and source, see on this link https Solve The Following Pairs Of Linear Equations By The Elimination Method And The Substitution Method Ii X 2 2y 3 1 And X Y 3 3About Elimination Use elimination when you are solving a system of equations and you can quickly eliminate one variable by adding or subtracting your equations together You can use this Elimination Calculator to practice solving systems




Solve 2 X 2 3y 1 6 And 3 X 2 Y 0 And Hence Find A For




Solve Equations Using Substitution Method 2x Y 3 And 4x Y 3
Solve the following pair of linear equations by elimination method xy=5 and 2x3y=4 Solve the following pairs of equation by elimination method x y = 6 2x 3y = 4 Solve the above equations by method of elimination x – y = 1 and 5x – 3y = 1Solve the system by the elimination method 2x y 6 = 0 2x y 8 = 0 When you eliminate x, what is the resulting equation? SOLVING SYSTEMS OF EQUATIONS ALGEBRAICALLY How to Use the Elimination Method How to Use the Substitution Method 97 2 x 1 5 x ≥ 0 4 4 4 Simplify both sides of the equation x=3 Substitute 3 in place of x in one of the original equations y = 2x y=2 3 y=6 The solution of the system is (3,6)Modeling Solve the following systems of equations by elimination




2 X 2 3y 1 6 And 3 X 2 Y 0 Problem Set 1 Q6 1 Of Linear Equation In Two Variables Youtube



1
Solve equations using substitution method 2 x − y = 3 and 4 x y = 3 A − 1 and 1 B − 2 and 1 C 1 and − 1 D − 1 and − 1 Medium Answer Correct option is C 1 and − 1 2 x − y = 3 (1) 4 x y = 3 (2) From equation 1 y = 2 x − 3 Substitute the value of y in equation 2 4 x y =The elimination method for solving systems of linear equations uses the addition property of equality You can add the same value to each side of an equation So if you have a system x – 6 = −6 and x y = 8, you can add x y to the left side of the first equation and add 8 to the right side of the equation And since x y = 8, you are adding the same value to each side of the first The Elimination Method In this section, the goal is to review another completely algebraic method for solving a system of linear equations called the elimination method 13 or addition method 14This method depends on the addition property of equations 15 given algebraic expressions A, B, C, and D we have \(\text{If} \A = B \text { and } C = D , \text { then } A C = B



How To Solve The Following Simultaneous Equations By Elimination Method A 2x 3y 12 X Y 1 B 3x Y 10 C X 3y 1 3x 2y 4 0 D 5 Quora



By The Substitution Method Solve The Linear Equation That Is 8 X 5 Y Is Equal To 9 And 3 X 2 Y Is Equal To 4 Mathematics Topperlearning Com Usvpr6ll
Solve this linear system using the elimination method 3x – y = 3 x y = 17 Good heavens, the y's are already lined up and signed up for us to eliminate them (3x x) (y y) = (3 17) 4x = x = 5 Plug x = 5 into the second original equation and solve for y 5 y = 17 y = 12 The solution seems to be (5, 12) Let's make a quick check for body doubles, evil clones, or demonicSolve each of the following systems of equations by the method of crossmultiplication x 2y 1 = 0 2x 3y 12 = 0 asked Apr 26 in Linear Equations by Haifa ( Transcript Example 7 Solve the following pair of equations by substitution method 7x – 15y = 2 x 2y = 3 7x – 15y = 2 x 2y = 3 From (1) 7x – 15y = 2 7x = 2 15y x = (𝟐 𝟏𝟓𝒚)/𝟕 Substituting the value of x in (2) x 2y = 3 (2 15𝑦)/7 2𝑦=3 Multiplying both sides by 7 7 × ((2 15𝑦)/7) 7×2𝑦=7×3 (2 15y) 14y = 21 15y 14y = 21 – 2 29y = 21 – 2
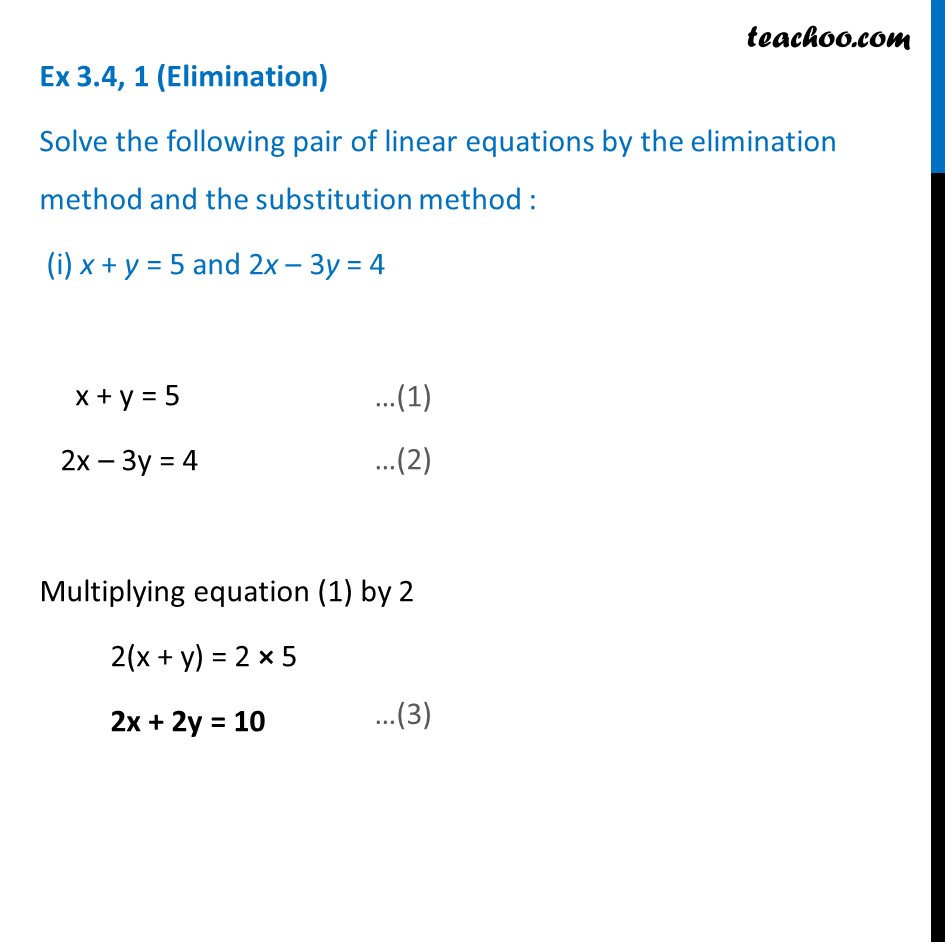



Ex 3 4 1 Solve By Elimination And Substitution I X Y 5 2x 3y
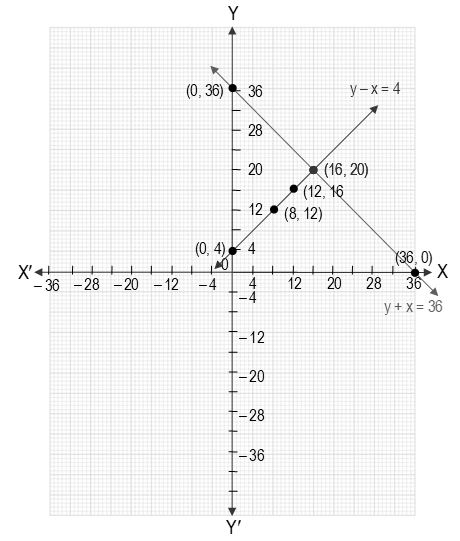



Pair Of Linear Equations In Two Variables
Solving Linear Equations by Elimination Method Here we are going to see some example problems of solving linear equations in two variables using elimination method The various steps involved in the technique are given below Step 1 Multiply one or both of the equations by a suitable number (s) so that either the coefficients of first Solve for x and y 3/x 6/y = 7, 9/x 3/y = 11 asked Jun 23 in Linear Equations by Hailley (334k points) linear equations in two variables;Solve by Addition/Elimination xy=1 xy=3 Multiply each equation by the value that makes the coefficients of opposite Simplify Multiply by Multiply by Multiply by Add the two equations together to eliminate from the system Divide each term by and simplify Tap for more steps Divide each term in by Cancel the common factor of




How Do You Solve X 2 Y 3 6 And X 3 Y 2 12 Socratic
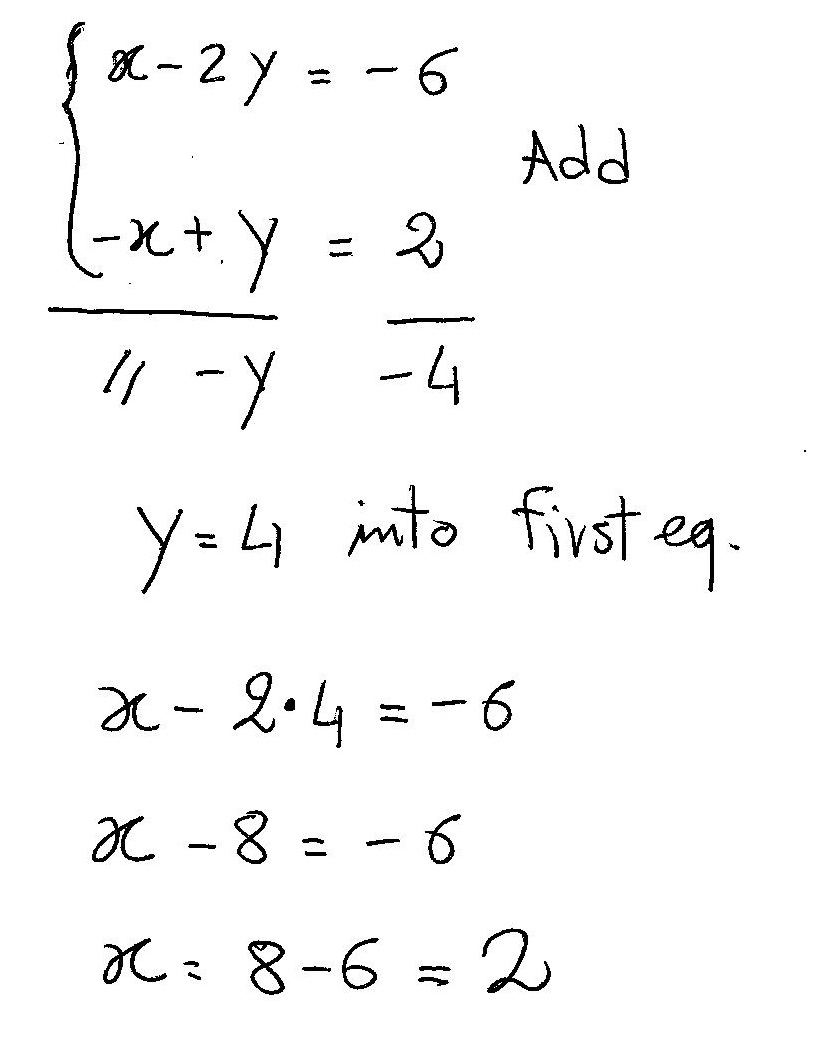



How Do You Solve X 2y 6 And X Y 2 Socratic
Correct answer Do equation by elimination method x_3y_4=6 2nd equation x_6y_2=6 eanswersincom Explanation You must make an equation that has only one variable in it so you can solve for that variable By finding the variable you can use it to find the other one Let's solve for y x y = 3 → y = 3 −x substitute (3 −x) instead of y in x = 3y −5 we get x =Solve the Given equation in Elimination method and Substitution Method



Solve 5 X 1 1 Y 2 2 And 6 X 1 3 Y 2 1 Studyrankersonline




3 Systems Of Linear Equations
OSMBOY OSMBOY Math Secondary School answered X/22y/3=1 and xy/3=3 solve by elimination method 2 See answers KrishnaPolavarapu KrishnaPolavarapu Here's your answer Hope it was helpful X/22y/3=1 and xy/3=3 solve by elimination method Get the answers you need, now!👍 Correct answer to the question 2) Use the elimination method to solve for x and y equation 1 x/3 y/6 = 4 equation 2 x/6 y/2 = 9 solve for y solve for x eeduanswerscom




Graph Graph Inequalities With Step By Step Math Problem Solver




Solve The Following System Of Equations By Using The Method Of
X 8y = 42 By signing up, you'll get thousands of stepbystep solutions to your homework👍 Correct answer to the question 2) Use the elimination method to solve for x and y equation 1 x/3 y/6 = 4 equation 2 x/6 y/2 = 9 solve for y solve for x ehomeworkhelpercom(a) 2x 3y = 12(i) and x y = 1(ii) (ii)×3 ==> 3x 3y = 3(iii) Now we can eliminate y by adding (i) & (iii) (i) (iii) ==> 5x = 15 so x=3
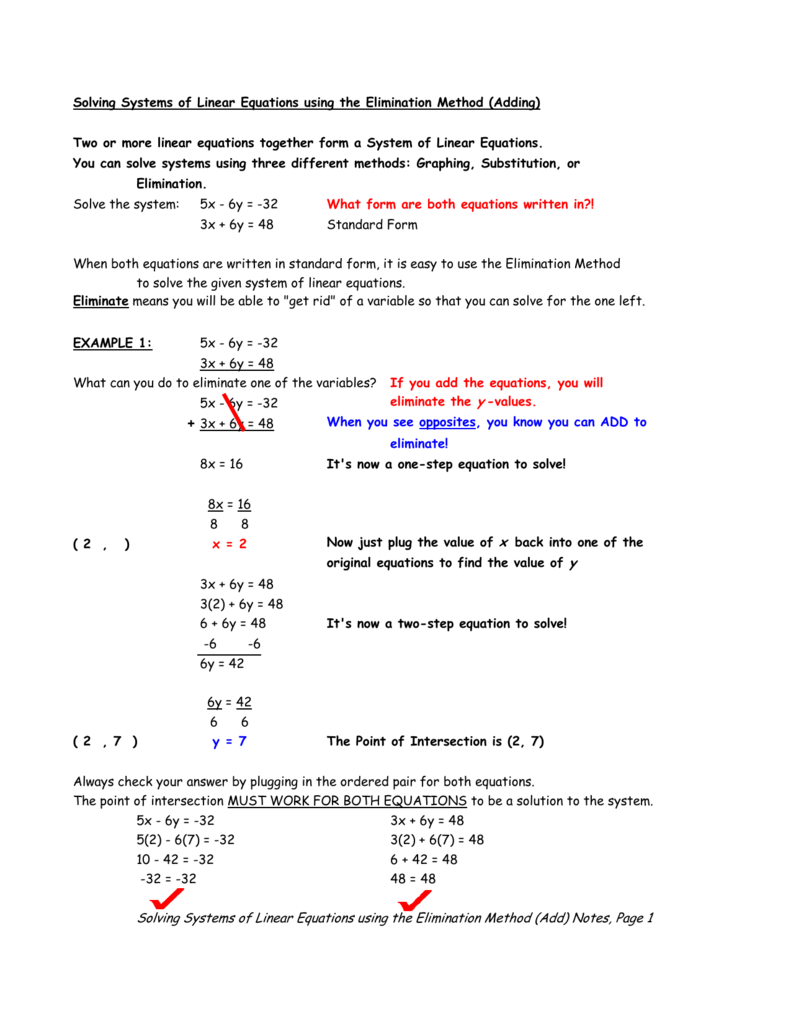



Solving Systems Of Linear Equations Using The Elimination Method




X Y 6 X Y 2 By Elimination Method Novocom Top
Free PreAlgebra, Algebra, Trigonometry, Calculus, Geometry, Statistics and Chemistry calculators stepbystepFree system of equations calculator solve system of equations stepbystepElimination Method Steps Step 1 Firstly, multiply both the given equations by some suitable nonzero constants to make the coefficients of any one of the variables (either x or y) numerically equal Step 2 After that, add or subtract one equation from the other in such a way that one variable gets eliminatedNow, if you get an equation in one variable, go to Step 3
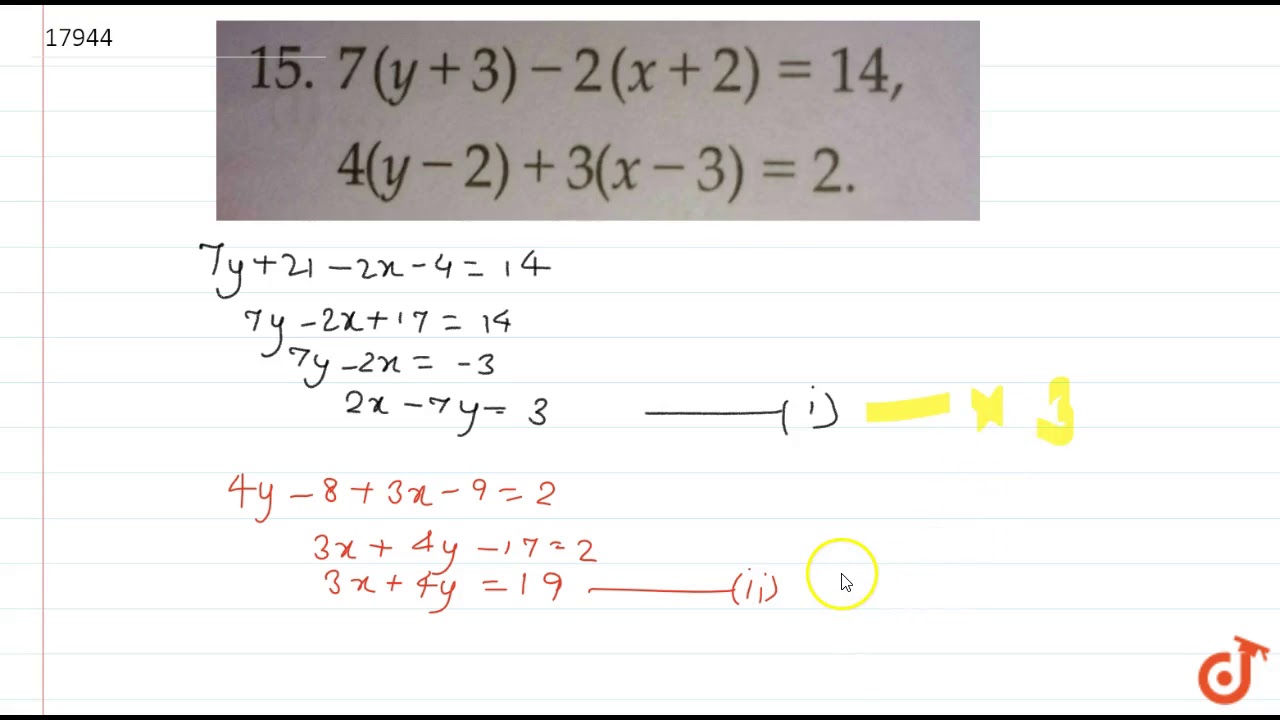



7 Y 3 2 X 2 14 4 Y 2 3 X 3 2 Youtube




4x X 8 Y 8 17 2y X 5y 3 2 Solve By Elimination Maths Simultaneous Linear Equations Meritnation Com
Xy=1 X_y=3 In Elimination Method 6,351 results, page 6 Algebra Solve by elimination 4x 9y = 9 x 3y = 6 algebra Explain the difference between solving a system of equations by the algebraic method and the graphical method Someone also wants to know why there are different methods for solving the same problemwhat would you tell him?Use elimination to solve each system of equations xy=3 2x3y=16 Gauss jordan elimination Use Gauss jordan elimination to solve for the values of the unknown x yz = 2x 3y z = 15 3x 5y 2z = 10 math I need help on this math problem Solve the systems of equations by elimination 2xy=7 6x12y=24 mathX 2 2y 3 1 And X Y 3 3 Find X And Y Values Using Elimination And Substitution Method Youtube Solve The Following Pairs Of Linear Equations By The Elimination Method And The Substitution Method Ii X 2 2y 3 1 And X Y 3 3 Sarthaks Econnect Largest Online Education Community For more information and source,




X Y 5 2x 2y 10 Elimination Method Novocom Top



Solved Find The Common Solution Of Each Using The Elimination Substitution And Graphical Method 2x Y 2 X 3y 36 2 3x Y 6 X Y 6 3 Course Hero
Solution Step 1 Select a variable which you want to eliminate from the equations Let us select y y 4x−3y = 32 xy = 1 4 x − 3 y = 32 x y = 1 Step 2 Take suitable constants and multiply them with the given equations so as to make the coefficients of the variable (which we want to eliminateElimination Method Solve for x and y by Elemination Method Class 10 maths ch 3 linear equation in Two VariablesHow to Solve X and Y By Elimination MethodSolve by the elimination method 3x y = 3 x 3y = 31 Get more help from Chegg Get 11 help now from expert Algebra tutors Solve it with our algebra




X 2 2y 3 1 And X Y 3 3 By Elimination Method Novocom Top




Solve X Y 3 And X 3 Y 2 6 By Substituting Methos Scholr




6 X Y 7 X Y 3 1 2 X Y 1 3 X Y



How To Transform The Linear Equations X Y Z 6 2x Y 2z 10 3x 3y 4z 21 In A Matrix Equation And Solve Them By Finding The Inverse Of Coefficient Matrix Using The Adjoint Method Quora




X 2 2y 3 1 And X Y 3 3 Find X And Y Values Using Elimination And Substitution Method Youtube




Solve For X And Y 15 X Y 22 X Y 5 40 X Y 55 X Y 13 X Y And X Y




Chapter 5 Review Exercises




Chapter 3 Systems Of Linear Equations
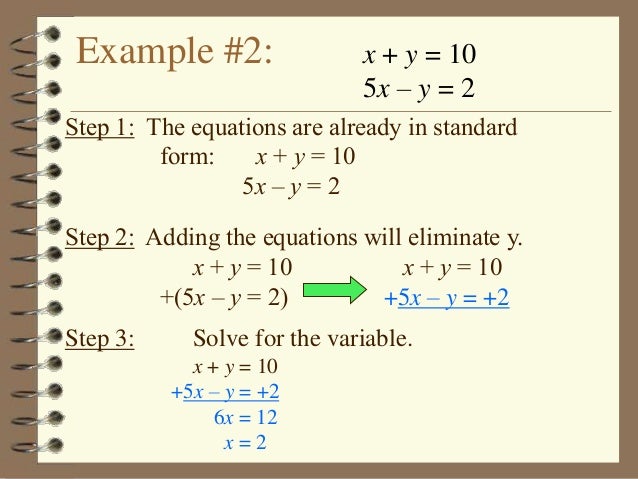



3 2 Solving Systems Of Equations Elimination Method
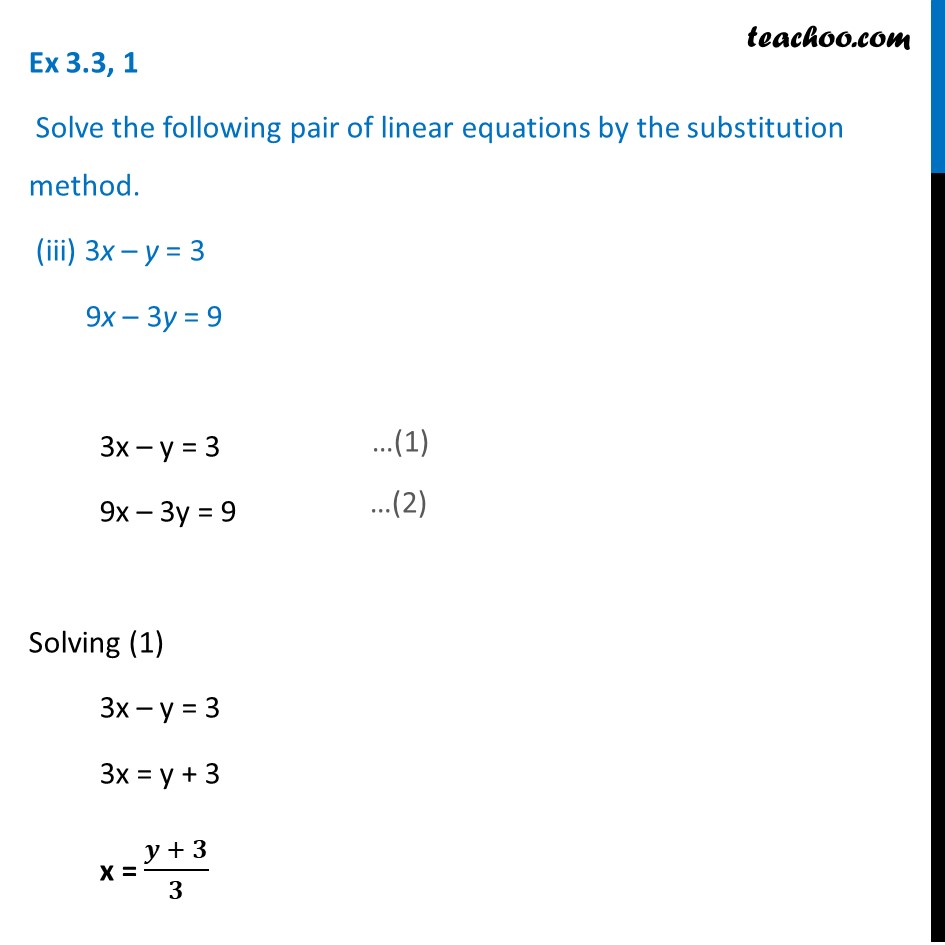



Ex 3 3 1 Solve By Substitution Method I X Y 14 Ex 3 3
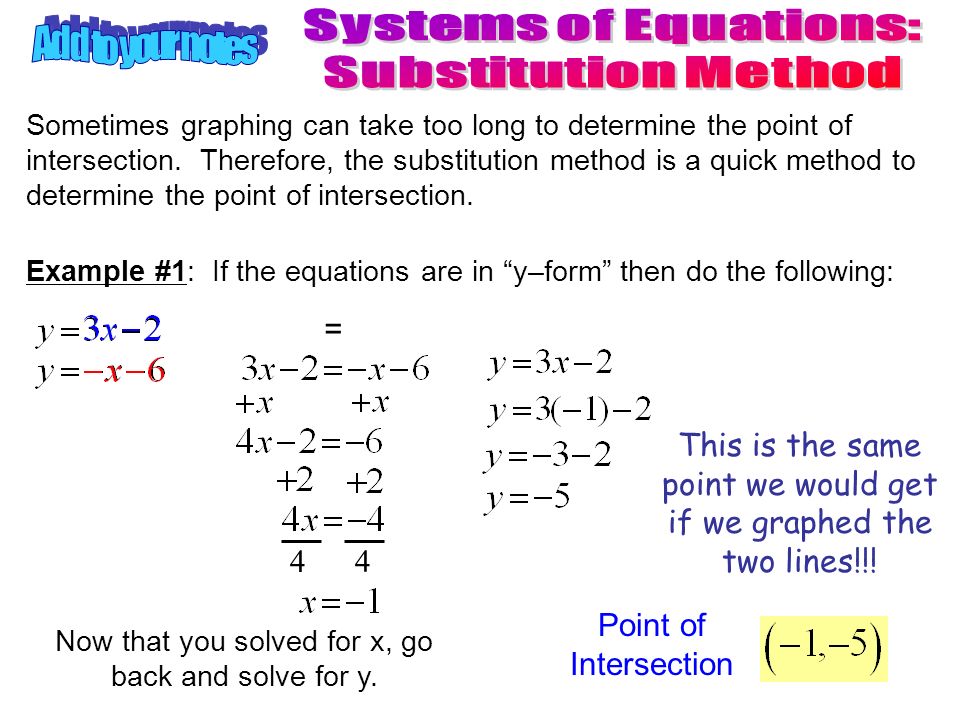



Systems Of Equations Substitution Method Ppt Video Online Download




Nm Tutorial Problems




How Do You Solve The System 4x 2y 6 And X Y 3 Socratic




Lo I Can Solve Simultaneous Equations Date




Solve The Following Pair Of Linear Equations By The Elimination M




Solve The Following System By Gauss Elimination Method M3 Notes Question Answer Collection




Warm Up Solve Each Equation For X 1 Y X Y 3x 4 Ppt Video Online Download




X Y 6 X Y 2 By Elimination Method Novocom Top



How Do You Solve The System 2 X 4 Y 6 3x 2 Y 3 13 Socratic



How To Transform The Linear Equations X Y Z 6 2x Y 2z 10 3x 3y 4z 21 In A Matrix Equation And Solve Them By Finding The Inverse Of Coefficient Matrix Using The Adjoint Method Quora




X Y 3 X 3 Y 2 6




2 Use Cramer S Rule To Solve The Following Set Of Chegg Com




Algebraic Elimination Method Class 10 Ncert Solutions Suresolv



Solve The Following Systems Of Equations 6 X Y 7 X Y 3 1 2 X Y 1 A X Y Where X Y 0 And X Y 0 Sarthaks Econnect Largest Online Education Community




X Y 3 And X 3 Y 2 6 Solve The Following Pair Of Linear Equations By The Elimination Method And Brainly In



Systems Of Linear Equations




3x 2 5y 3 2 And X 3 Y 2 13 6 Solve Using Substitution Method Youtube
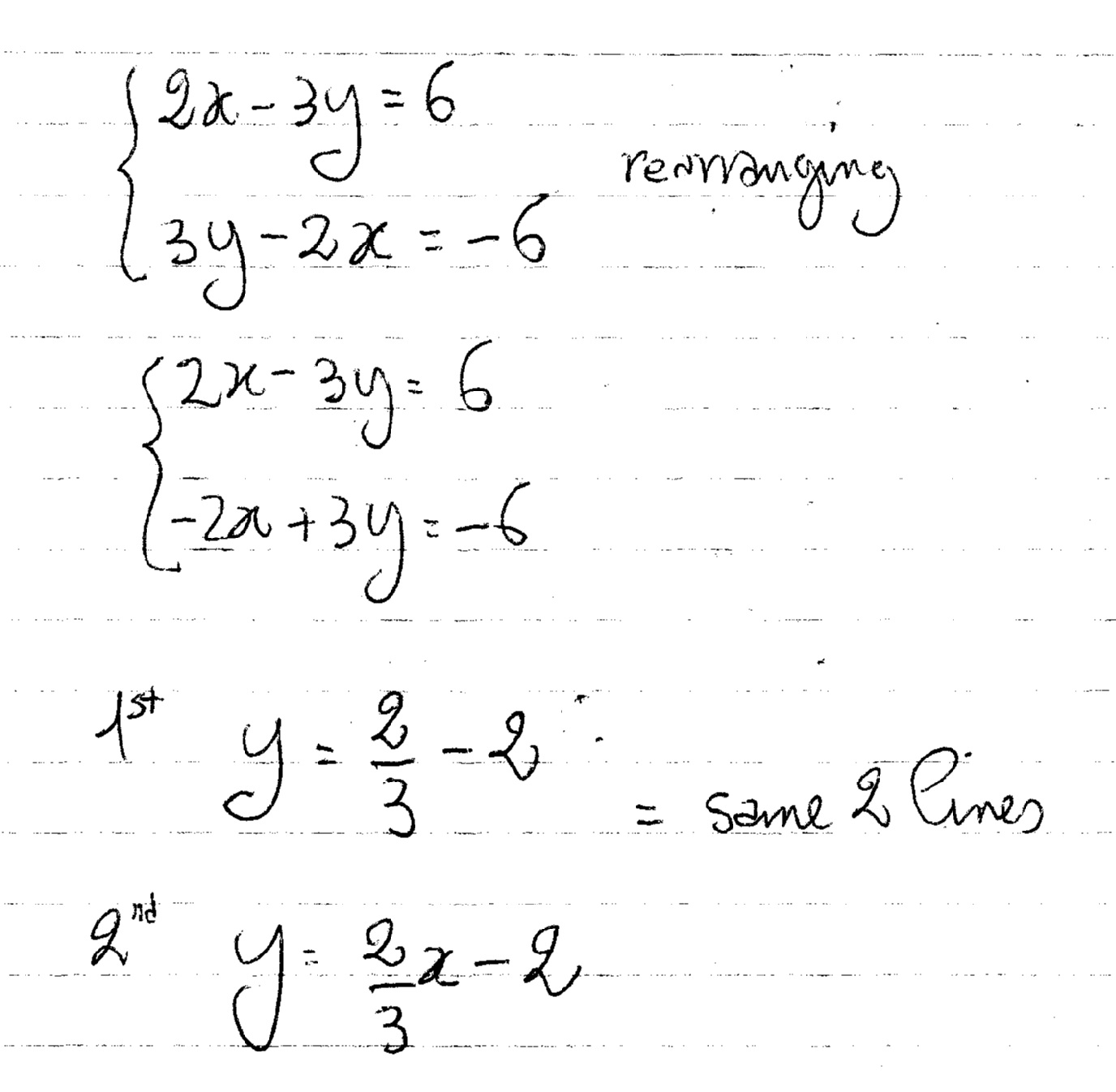



How Do You Solve The System Of Equations 2x 3y 6 And 3y 2x 6 Socratic




X 2y 1 X 4y 5 See How To Solve It At Qanda



1




Solve For X And Y X 6y 6 3x 8y 5




Lo I Can Solve Simultaneous Equations Date



Systems Of Linear Equations Graphing




Solving Simultaneous Equations The Substitution Method And The Addition Method Algebra Reference Electronics Textbook



Simultaneous Equations Gmat Math Study Guide




Rd Sharma Class 10 Solutions Maths Chapter 3 Pair Of Linear Equations In Two Variables Exercise 3 3




Systems Of Equations Substitution Method Ppt Video Online Download




Solve By Reducing Them To A Pair Of Linear Equations 5 X 1 1 Y 2 2 6 X 1 3 Y 2 1 Youtube




Solving Linear Systems




Solve The Following Pair Of Linear Elimination Method And The Subs
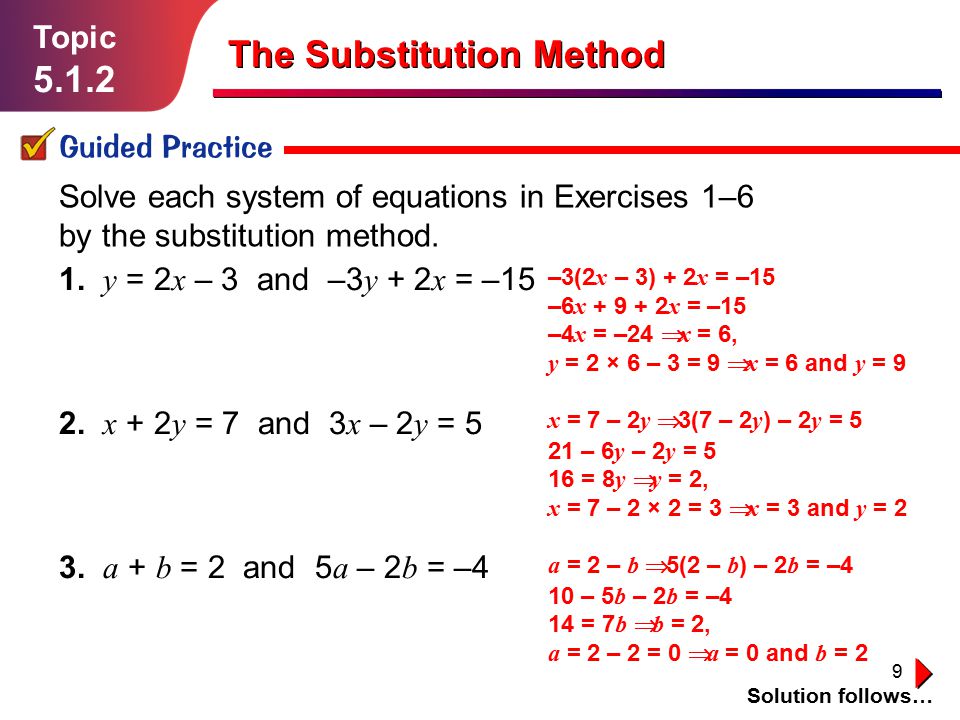



1 Topic The Substitution Method 2 Topic The Substitution Method California Standard 9 0 Students Solve A System Of Two Linear Equations Ppt Download
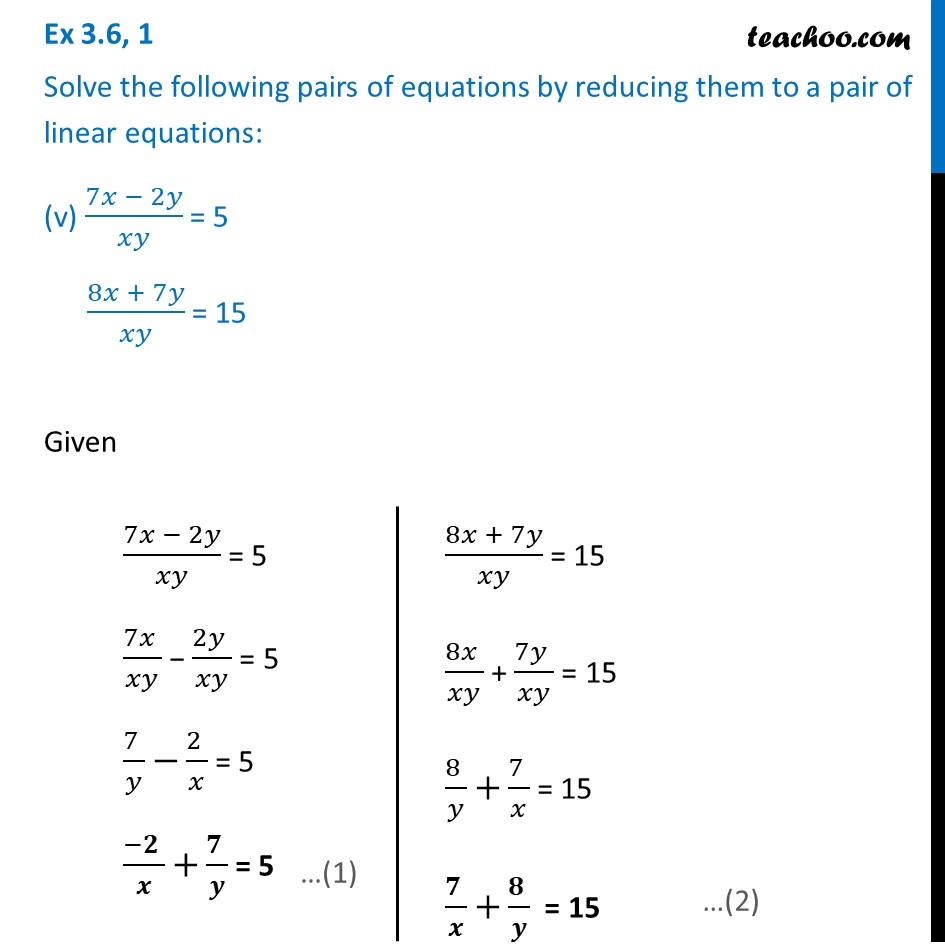



Ex 3 6 1 V And Vi 7x 2y Xy 5 8x 7y Xy 15




Solve The Following System Of Equations Sqrt 2 X Sqrt 3 Y 0




X Y 3 X 3 Y 2 6 Youtube
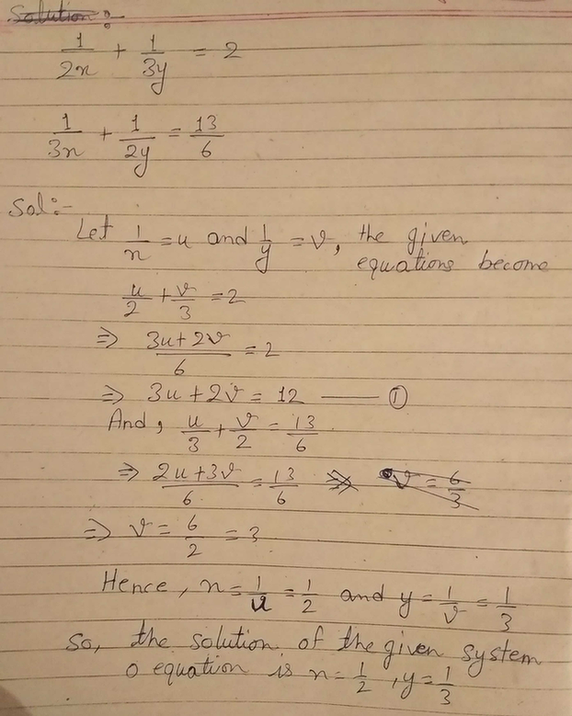



Exercise 3 5 1 Solve The Following Pairs Of Equations By Re Scholr
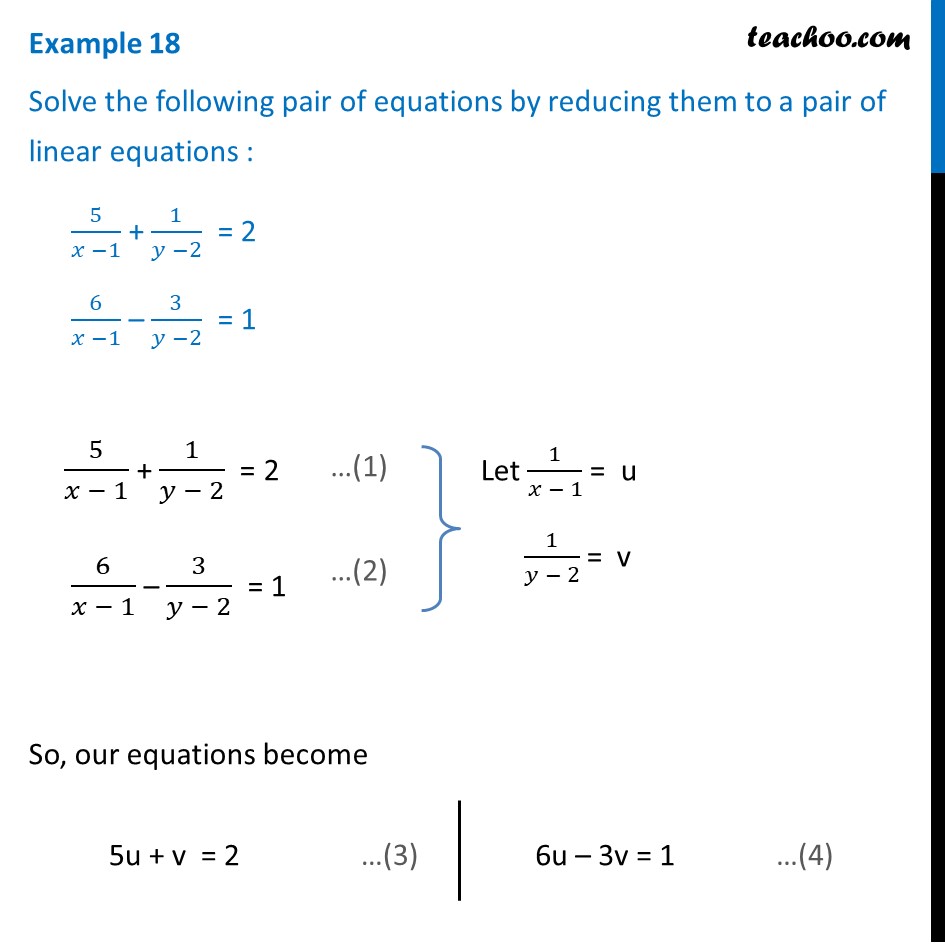



Example 18 Solve 5 X 1 1 Y 2 2 6 X 1 3 Y 2 1 Examples




X Y 3 X 3 Y 2 6




Consider The Following System Of Linear Equations X Chegg Com




Solve The Following System Of Equations 27 X Y 15 X Y 2 And 30 X Y 1 X Y 3 Mathematics Topperlearning Com X68shsoo




Solving A System Of Equations Using A Matrix Precalculus Socratic




Solution Of X Y 3 And X 3 Y 2 6 By Elimination Method Brainly In




If X Y 6 And 3x Y 4 Then What Is X Y Equal To Quora



Using Matrix Method Solve The System Of Equations 3x 2y 2z 3 X 2y 3z 6 2x Y Z 2 Studyrankersonline



The Elimination Method




Solution Of X Y 3 And X 3 Y 2 6 By Elimination Method Brainly In




Solved X 7 Y 3 5 X 2 Y 9 6 By Elimination Method Brainly In
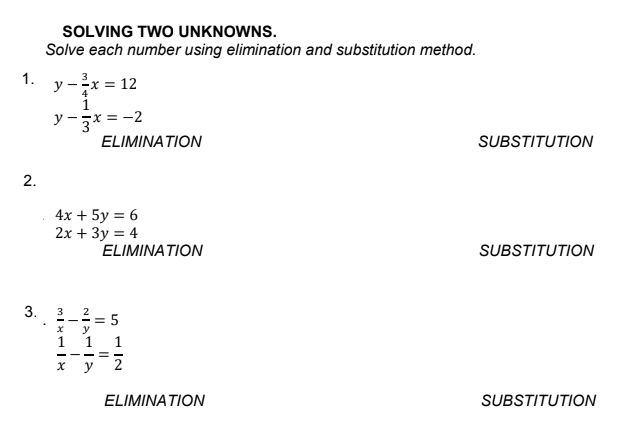



Answered Solve Each Number Using Elimination And Bartleby



What Are X And Y From The System Equation 2x 3y 4 X Y 6 Quora




22 4 3x 6 317 3 2x 3y 11 4 X 2y 8 6 26 Chegg Com



Solve The Following Pairs Of Linear Equations By The Substitution Method 3x 2 5y 3 2 X 3 Y 2 13 6 Sarthaks Econnect Largest Online Education Community
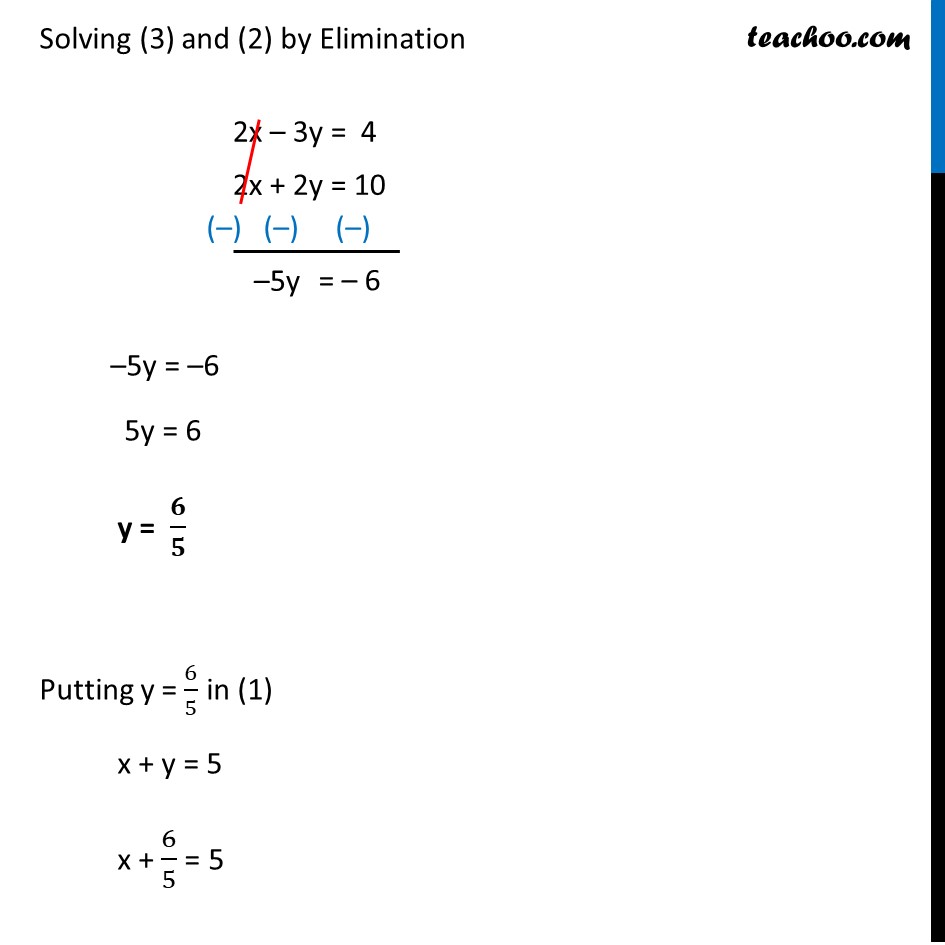



Ex 3 4 1 Solve By Elimination And Substitution I X Y 5 2x 3y




Solve For X And Y 40 X Y 2 X Y 5 And 25 X Y 3 X Y 1 Mathematics Topperlearning Com Idbi1itt




X Y 6 X Y 2 By Elimination Method Novocom Top




X 2 2y 3 1 And X Y 3 3 Solve By Elimination Method Brainly In




6 2 Solving Systems Of Linear Equations 455 We Have Chegg Com



1




X 7 Y 3 5 X 2 Y 9 6 By Subsitution Method Brainly In
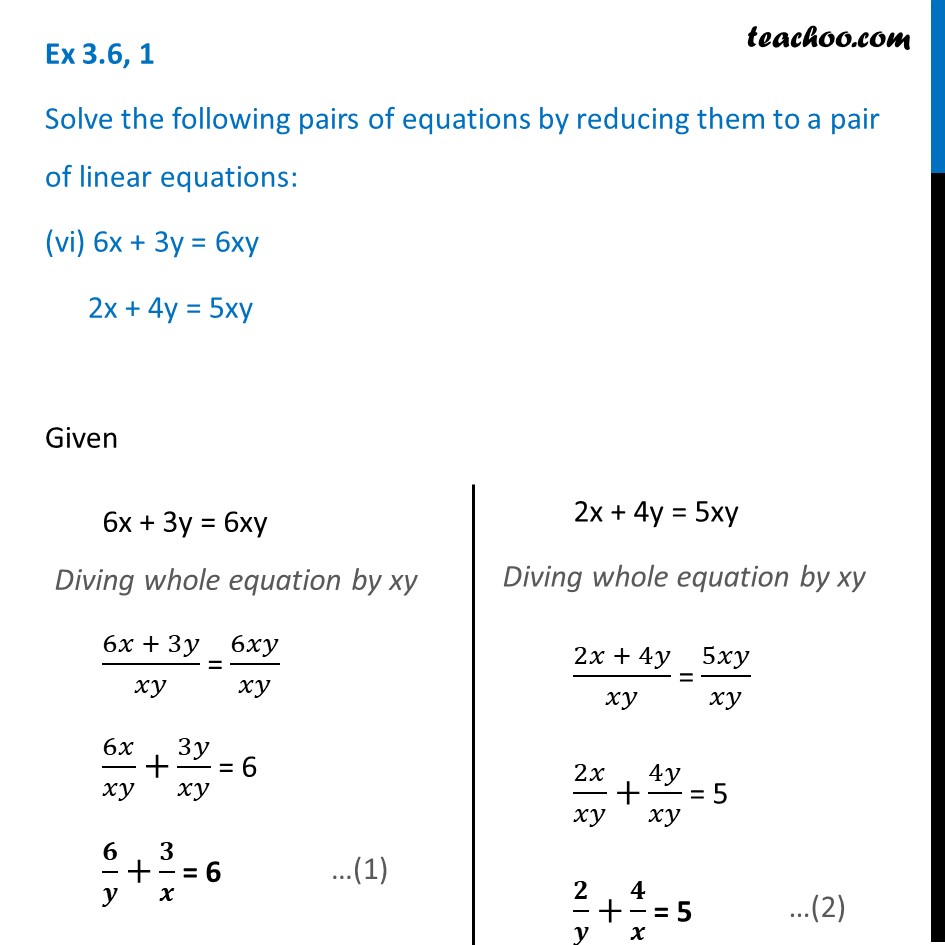



Ex 3 6 1 V And Vi 7x 2y Xy 5 8x 7y Xy 15




3x 2 5y 3 2 And X 3 Y 2 13 6 Solve Using Substitution Method Youtube




X Y 3 X 3 Y 2 6 Elimination Method Brainly In
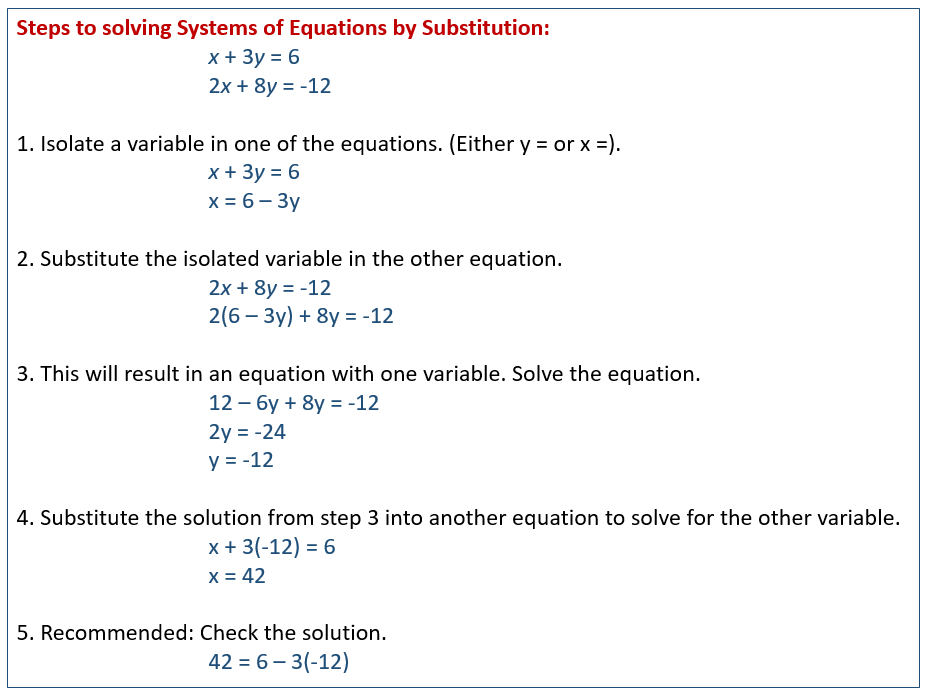



Solving Systems Of Equations With Fractions Or Decimals Video Lessons Examples And Solutions



Who Can Solve This X Y 15 And X Y 3 Find X And Y Quora




X 7 Y 3 5 X 2 Y 9 6 By Elimination Method Novocom Top



How To Solve This System Of Equations 3x 5y 6 2x 3y 4 Quora



1



Solve The Following Systems Of Equations 6 X Y 7 X Y 3 1 2 X Y 1 A X Y Where X Y 0 And X Y 0 Sarthaks Econnect Largest Online Education Community
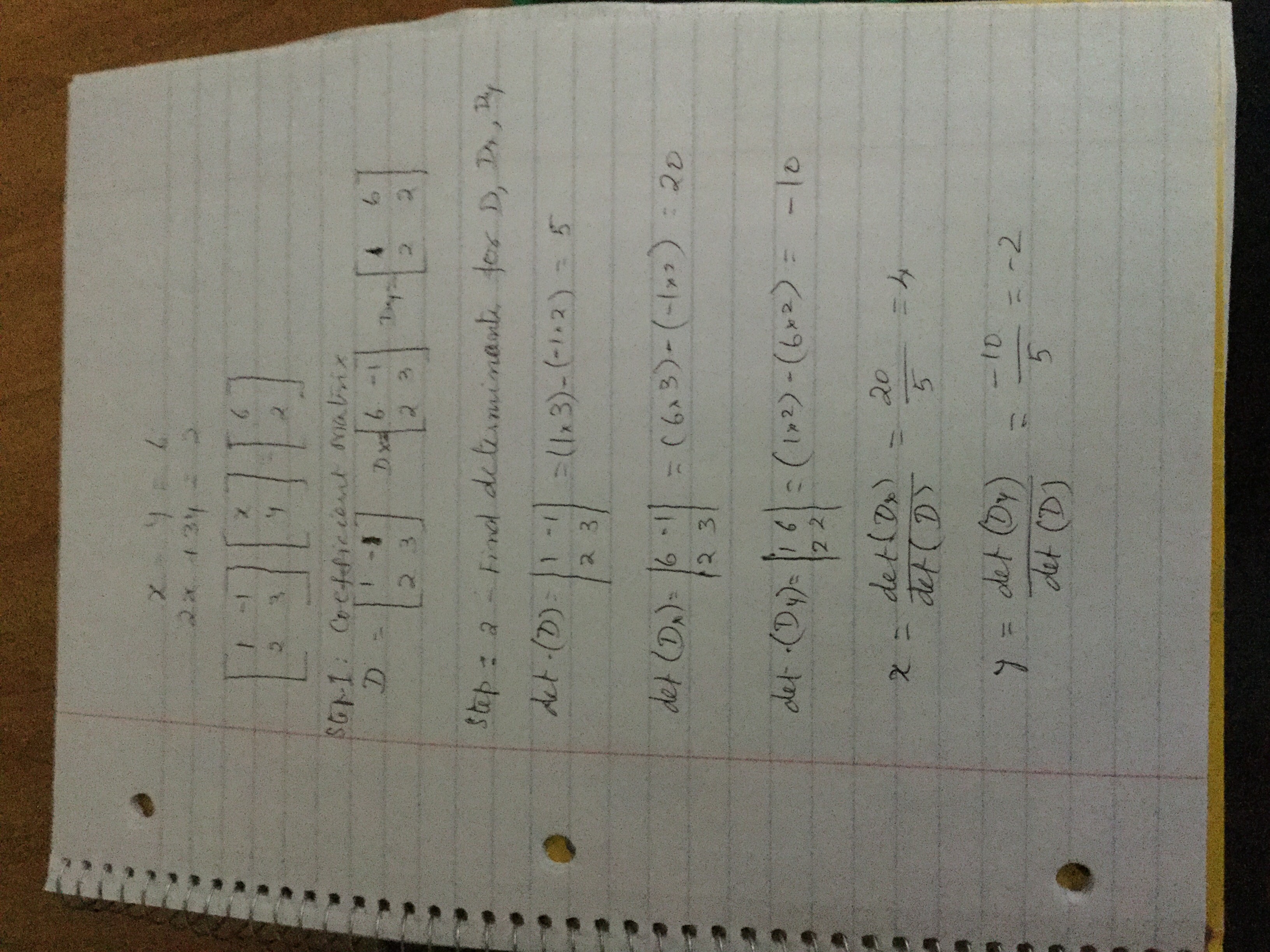



How Do You Solve X Y 6 And 2x 3y 2 Using Matrices Socratic




Example 12 Use Elimination Method 2x 3y 8 4x 6y 7



No comments:
Post a Comment